Sangaku - first inspiration
Apart from the trip to Hong Kong, one of the most inspiring things that I have learned from is some small boards on which my teacher usually wrote some problems. Every day, my head teacher came to the end of the class, hang up on a board with a small math question and prize, sometimes a ruler, rubber, or even a pencil. That depended a lot on the difficulty of the question.
Somehow years later, when I read a post on our Japanese cultural club, I realized that these tablets originated from Japan and are called sangaku. In the 7th century, Japan started the closed country policy, and teachers, who are retired Samurai, invented the so-called sangaku. The first sangaku to be printed and translated into English in the Scientific American magazine was undertaken by Hidetoshi and Daniel in 1989.
Although the wooden boards that my teacher hung up that year were not any well-known problems, it was still one of the things that I always looked forward to when the bell rang. Maybe I did not have enough rulers, pencils, or rubbers.
Let's have a look that one of the examples of Sangaku:
P1: Let a cyclic polygon be triangulated in any manner by non-intersecting diagonals. Prove that the sum of the inradii of the triangles formed is a constant independent of the triangulation chosen (invariant).
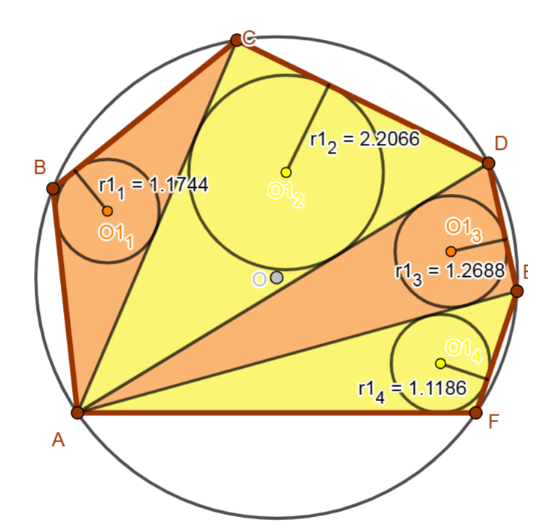
P2: Given a cyclic quadrilateral ABCD and let A1, B1, C1, D1 the incenters and ra, rb, rc, rd the inradii of the triangles BAD, ABC, BCD, ADC. Then (1) A1B1C1,D1 is a rectangle, (2) ra + rc = rb + rd

In a right triangle ABC (see the figure below) incircle O (radius r) is tangent to BC, AC, and AB at D, E, and F, respectively. Circle O1 (radius r1) is inscribed in quadrilateral AFOE and circle O2 (radius r2) is inscribed in quadrilateral CDOE. Prove that r.r = 2r1.r2.

The figure below shows the circles O1 (radius r1) and O2 (radius r2). A1A2 and B1B2 are the common external tangents and CD is a common internal tangent. Circle O3 (radius r3) touches A1C, CD, and circle O1. Circle O4 (radius r4) touches B1D, CD, and circle O1. Prove that:

